Geometry Properties of Equality: Key Concepts Explained
Understanding geometry properties of equality is essential for anyone studying mathematics or applying geometric principles in real-world scenarios. These properties form the foundation of geometric proofs and problem-solving, ensuring logical and consistent reasoning. Whether you’re a student, educator, or professional, mastering these concepts will enhance your mathematical skills and practical applications.
What Are Geometry Properties of Equality?
Geometry properties of equality are rules that govern the relationships between equal quantities in geometric figures. These properties allow us to manipulate equations, prove theorems, and solve problems systematically. Key properties include the reflexive property, symmetric property, transitive property, substitution property, and addition, subtraction, multiplication, and division properties.
📌 Note: Understanding these properties is crucial for constructing geometric proofs and solving complex problems.
Key Geometry Properties of Equality Explained

1. Reflexive Property
The reflexive property states that any quantity is equal to itself. Mathematically, if a is a real number, then a = a. This property serves as the basis for all other equality properties.
2. Symmetric Property
The symmetric property asserts that if a = b, then b = a. This property allows us to rearrange equations without changing their truth value.
3. Transitive Property
The transitive property states that if a = b and b = c, then a = c. This property is vital for chaining equalities in proofs.
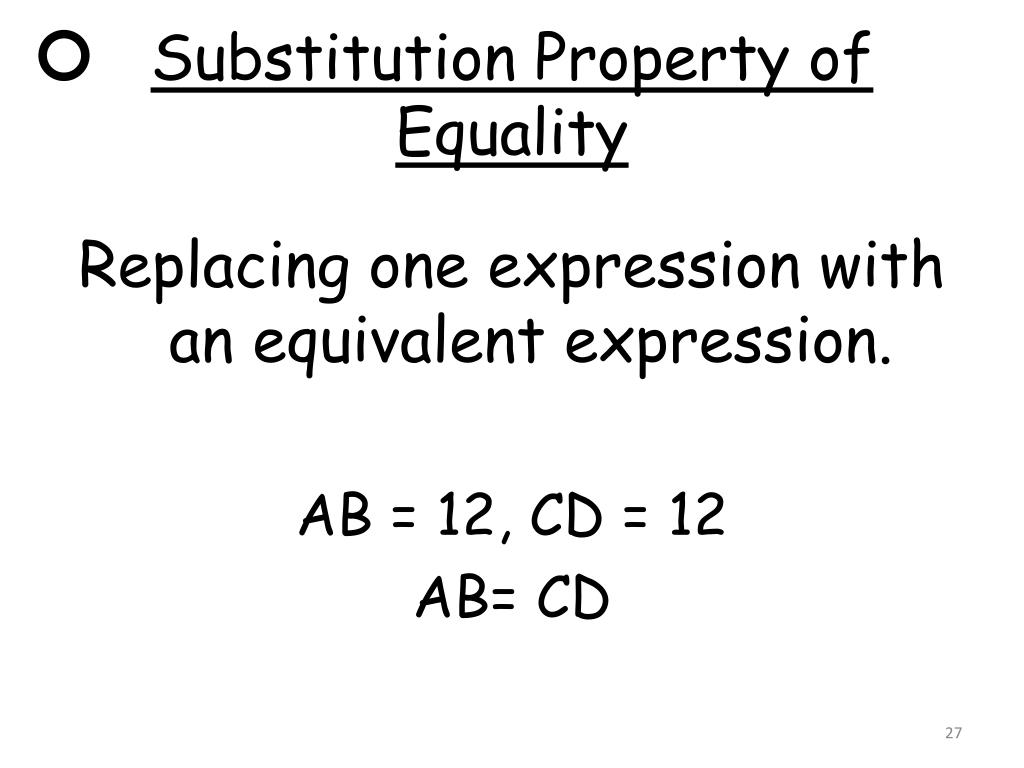
4. Substitution Property
The substitution property allows us to replace a variable with an equal quantity in any expression. For example, if a = b, then a can be substituted for b in any equation.
5. Addition, Subtraction, Multiplication, and Division Properties
These properties state that performing the same operation on both sides of an equation preserves equality. For instance, if a = b, then a + c = b + c, a - c = b - c, a × c = b × c, and a ÷ c = b ÷ c (assuming c ≠ 0).
| Property | Description |
|---|---|
| Reflexive | Any quantity is equal to itself. |
| Symmetric | If *a = b*, then *b = a*. |
| Transitive | If *a = b* and *b = c*, then *a = c*. |
| Substitution | Replace a variable with an equal quantity. |
| Addition/Subtraction/Multiplication/Division | Performing the same operation on both sides preserves equality. |

Practical Applications of Geometry Properties of Equality

These properties are not just theoretical; they have real-world applications in fields like engineering, architecture, and physics. For example, ensuring equal measurements in construction relies on the transitive property, while balancing equations in chemistry uses the substitution property.
💡 Note: Mastering these properties can simplify problem-solving in both academic and professional settings.
Checklist for Applying Geometry Properties of Equality

- Identify the given equalities in the problem.
- Use the reflexive property to establish base equalities.
- Apply the symmetric property to rearrange equations.
- Leverage the transitive property to chain equalities.
- Substitute equal quantities using the substitution property.
- Perform operations on both sides of the equation using addition, subtraction, multiplication, and division properties.
Wrapping Up

Mastering geometry properties of equality is fundamental to advancing in mathematics and its applications. By understanding and applying these properties, you can approach geometric problems with confidence and precision. Whether you’re proving theorems or solving real-world challenges, these rules will serve as your toolkit for success.
What is the reflexive property in geometry?
+The reflexive property states that any quantity is equal to itself. For example, if *a* is a real number, then *a = a*.
How does the transitive property help in geometric proofs?
+The transitive property allows you to chain equalities. If *a = b* and *b = c*, then *a = c*, which is essential for constructing logical proofs.
Can geometry properties of equality be applied outside mathematics?
+Yes, these properties are used in fields like engineering, architecture, and physics to ensure accuracy and consistency in measurements and equations.
Related Keywords: Geometry proofs, geometric theorems, mathematical properties, real-world geometry applications, problem-solving techniques.