Understanding Grand Canonical Ensemble in Thermal Hartree-Fock Theory
<!DOCTYPE html>
The Grand Canonical Ensemble (GCE) is a powerful tool in statistical mechanics, offering insights into systems with fluctuating particle numbers. When combined with Thermal Hartree-Fock Theory, it becomes a cornerstone for understanding many-body systems at finite temperatures. This blog post delves into the intricacies of GCE within the context of Thermal Hartree-Fock Theory, providing both informative and commercial insights for readers.
What is the Grand Canonical Ensemble?

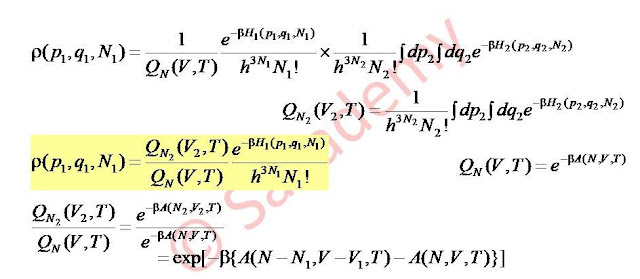
The Grand Canonical Ensemble describes a system that exchanges both energy and particles with a reservoir. It is characterized by the chemical potential ((\mu)), temperature ((T)), and volume ((V)). The grand partition function ((\mathcal{Z})) is central to GCE, encapsulating all thermodynamic properties of the system. (Statistical Mechanics,Thermodynamic Ensembles,Many-Body Physics)
Thermal Hartree-Fock Theory: A Brief Overview

Thermal Hartree-Fock (THF) Theory extends the Hartree-Fock approximation to finite temperatures. It accounts for thermal excitations, making it suitable for studying systems like nuclei, quantum dots, and ultracold atoms. THF combines mean-field theory with thermal Green’s functions, providing a robust framework for many-body systems. (Mean-Field Theory,Quantum Many-Body Systems,Finite Temperature Methods)
Integrating Grand Canonical Ensemble with Thermal Hartree-Fock Theory

Combining GCE with THF allows for a detailed study of systems with variable particle numbers at finite temperatures. This integration involves:
- Grand Potential ((\Omega)): Derived from the grand partition function, it governs the system’s thermodynamics.
- Chemical Potential ((\mu)): Controls particle exchange, crucial for open systems.
- Thermal Occupation Numbers: Determine the probability of particles occupying specific states.
📌 Note: The integration of GCE and THF is computationally intensive but yields precise results for complex systems.
Applications of GCE in Thermal Hartree-Fock Theory

This combined approach finds applications in:
- Nuclear Physics: Studying nuclei at finite temperatures and densities.
- Condensed Matter Physics: Investigating quantum dots and ultracold atoms.
- Astrophysics: Modeling neutron stars and supernovae.
(Nuclear Structure,Condensed Matter Systems,Astrophysical Phenomena)
Commercial Relevance: Tools and Software

For commercial-intent visitors, several software packages facilitate GCE and THF calculations:
| Software | Key Features |
|---|---|
| Sky3D | Solves THF equations in 3D systems. |
| HFODD | Focuses on deformed nuclei using THF. |
| Nuccini | Specialized for nuclear structure calculations. |

(Computational Physics,Scientific Software,Research Tools)
Checklist for Implementing GCE in THF
Follow these steps to effectively apply GCE in Thermal Hartree-Fock Theory:
- Define system parameters: (T), (V), and (\mu).
- Compute the grand partition function ((\mathcal{Z})).
- Derive the grand potential ((\Omega)) and thermal occupation numbers.
- Analyze thermodynamic properties using (\Omega).
(Thermodynamic Calculations,Many-Body Simulations,Statistical Mechanics)
In summary, the Grand Canonical Ensemble in Thermal Hartree-Fock Theory provides a comprehensive framework for studying many-body systems at finite temperatures. Whether for academic research or commercial applications, understanding this integration opens doors to advanced computational physics. By leveraging tools and following structured methodologies, researchers can unlock profound insights into complex systems.
What is the Grand Canonical Ensemble?
+The Grand Canonical Ensemble is a statistical mechanics framework for systems exchanging energy and particles with a reservoir, characterized by temperature, volume, and chemical potential.
How does Thermal Hartree-Fock Theory differ from standard Hartree-Fock?
+Thermal Hartree-Fock Theory extends the standard Hartree-Fock method to finite temperatures, incorporating thermal excitations and occupation numbers.
What are the main applications of GCE in THF?
+GCE in THF is applied in nuclear physics, condensed matter physics, and astrophysics to study systems like nuclei, quantum dots, and neutron stars.