Mastering Recursive Formulas: A Simple Step-by-Step Guide

Mastering recursive formulas is a crucial skill for anyone working with sequences, algorithms, or mathematical modeling. Whether you're a student, a programmer, or a data analyst, understanding how to create and manipulate recursive formulas can simplify complex problems and unlock new possibilities. In this guide, we’ll walk you through the basics, provide step-by-step instructions, and offer practical tips to help you master recursive formulas effectively. (recursive formulas, mathematical sequences, algorithm development)
What Are Recursive Formulas?

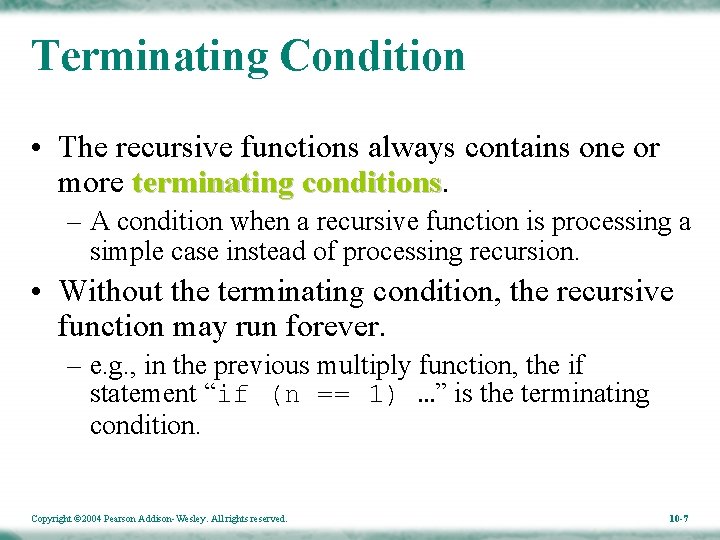
Recursive formulas define a sequence where each term is based on the previous one(s). Unlike explicit formulas, which directly compute a term, recursive formulas rely on a starting point (base case) and a rule to generate subsequent terms. This approach is widely used in mathematics, computer science, and finance. (sequence definition, base case, recursive rule)
Step 1: Understand the Base Case

Every recursive formula begins with a base case, which is the initial term(s) of the sequence. Without a base case, the formula cannot generate further terms. For example, in the Fibonacci sequence, the base cases are ( F(0) = 0 ) and ( F(1) = 1 ). (base case, Fibonacci sequence, initial term)
Step 2: Define the Recursive Rule

The recursive rule describes how to compute the next term using previous terms. For the Fibonacci sequence, the rule is ( F(n) = F(n-1) + F(n-2) ). This rule applies for all ( n \geq 2 ). (recursive rule, Fibonacci sequence, term calculation)
Step 3: Apply the Formula Iteratively

Once the base case and rule are defined, apply the formula iteratively to generate the sequence. For instance, to find ( F(3) ), use ( F(2) = F(1) + F(0) = 1 + 0 = 1 ), and then ( F(3) = F(2) + F(1) = 1 + 1 = 2 ). (iterative application, sequence generation, term calculation)
📌 Note: Always ensure the base case is correctly defined, as errors here will propagate through the entire sequence.
Practical Tips for Mastering Recursive Formulas

- Start Simple: Practice with basic sequences like arithmetic or geometric progressions before moving to complex ones.
- Use Visual Aids: Draw flowcharts or diagrams to visualize how terms are generated.
- Test Your Formula: Verify your formula by manually computing the first few terms and comparing them to known values.
- Optimize for Efficiency: In programming, be mindful of recursion depth to avoid stack overflow errors.
| Sequence | Base Case | Recursive Rule |
|---|---|---|
| Fibonacci | F(0) = 0, F(1) = 1 | F(n) = F(n-1) + F(n-2) |
| Arithmetic | a_0 = c | a_n = a_{n-1} + d |
Checklist for Mastering Recursive Formulas
- Define the base case clearly.
- Write the recursive rule accurately.
- Test the formula with small values.
- Optimize for computational efficiency.
- Practice with diverse sequence types.
Mastering recursive formulas opens up a world of possibilities in mathematics and programming. By understanding the base case, defining the recursive rule, and applying the formula iteratively, you can tackle complex problems with ease. Remember to practice regularly and use visual aids to solidify your understanding. (recursive formulas, mathematical modeling, programming efficiency)
What is a recursive formula?
+A recursive formula defines a sequence where each term is based on the previous term(s), starting from a base case.
Why is the base case important?
+The base case provides the initial term(s) of the sequence, without which the recursive rule cannot generate further terms.
How can I optimize recursive formulas in programming?
+Use techniques like memoization to store previously computed values and avoid redundant calculations.



