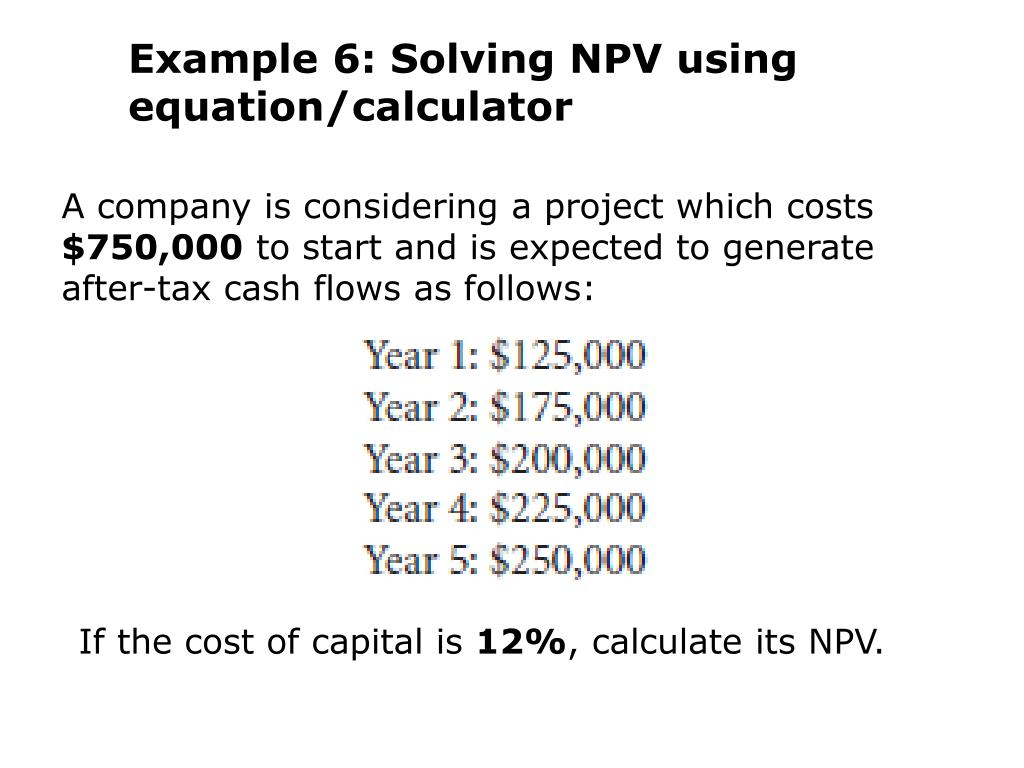
Mutually Exclusive vs Independent: Key Differences Explained

Understanding the concepts of mutually exclusive and independent events is crucial in probability and statistics. These terms often appear in data analysis, decision-making, and risk assessment. While they might seem similar, they describe fundamentally different relationships between events. This blog post will clarify their definitions, key differences, and practical applications, ensuring you can apply these concepts effectively.
What Are Mutually Exclusive Events?

Mutually exclusive events are events that cannot occur at the same time. If one event happens, the other cannot. For example, flipping a coin cannot result in both heads and tails simultaneously.
Key Characteristics of Mutually Exclusive Events
- Cannot coexist: The occurrence of one event prevents the other.
- Probability addition rule: The probability of either event occurring is the sum of their individual probabilities.
- Examples: Rolling a 1 and rolling a 6 on a single die, selecting a king and selecting a queen from a deck of cards.
📌 Note: Mutually exclusive events are often confused with independent events, but they are distinct concepts.
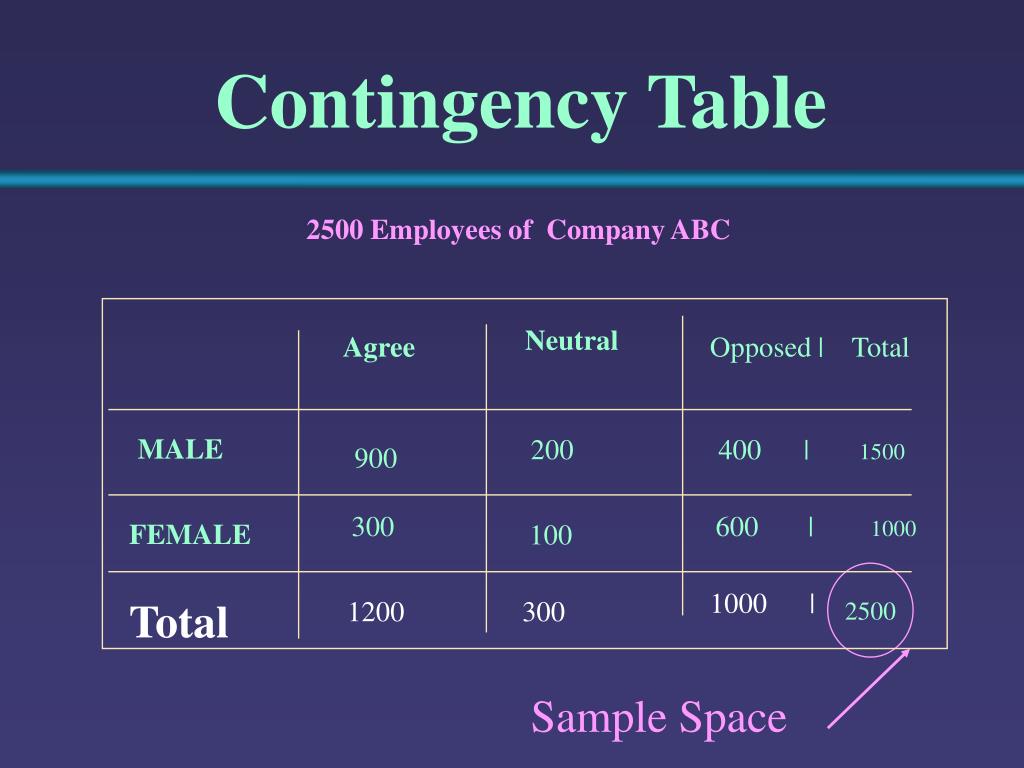
What Are Independent Events?
Independent events are events where the occurrence of one does not affect the probability of the other. The outcome of one event has no influence on the outcome of the other. For instance, flipping a coin twice results in independent events.
Key Characteristics of Independent Events
- No influence: The probability of one event remains unchanged regardless of the other.
- Probability multiplication rule: The probability of both events occurring is the product of their individual probabilities.
- Examples: Flipping a coin and rolling a die, selecting a card from a deck and spinning a roulette wheel.
Mutually Exclusive vs Independent: Key Differences

To better understand the contrast, let’s compare these concepts side by side:
| Aspect | Mutually Exclusive | Independent |
|---|---|---|
| Coexistence | Cannot occur together | Can occur together |
| Probability Rule | Addition | Multiplication |
| Example | Heads and tails in a single coin flip | Two consecutive coin flips |
Practical Applications

Understanding these concepts is essential in various fields:
- Finance: Assessing risks in investments.
- Marketing: Analyzing customer behavior.
- Healthcare: Evaluating treatment outcomes.
Checklist: Mutually Exclusive vs Independent

- Mutually Exclusive:
- Can both events occur simultaneously? If no, they are mutually exclusive.
- Use the addition rule for probabilities.
- Can both events occur simultaneously? If no, they are mutually exclusive.
- Independent:
- Does one event affect the probability of the other? If no, they are independent.
- Use the multiplication rule for probabilities.
- Does one event affect the probability of the other? If no, they are independent.
Wrapping Up
In summary, mutually exclusive events cannot happen together, while independent events do not influence each other’s probabilities. Recognizing these differences is vital for accurate data analysis and decision-making. Whether you’re a student, professional, or enthusiast, mastering these concepts will enhance your understanding of probability and statistics.
Can two events be both mutually exclusive and independent?
+No, mutually exclusive events cannot be independent because the occurrence of one directly affects the probability of the other.
How do you calculate the probability of mutually exclusive events?
+Add the probabilities of each individual event to find the probability of either occurring.
What is an example of independent events in real life?
+Flipping a coin and rolling a die are independent events because the outcome of one does not affect the other.
probability theory,statistics,data analysis,decision-making,risk assessment



