Mastering Geometry: Essential Properties of Equalities Explained
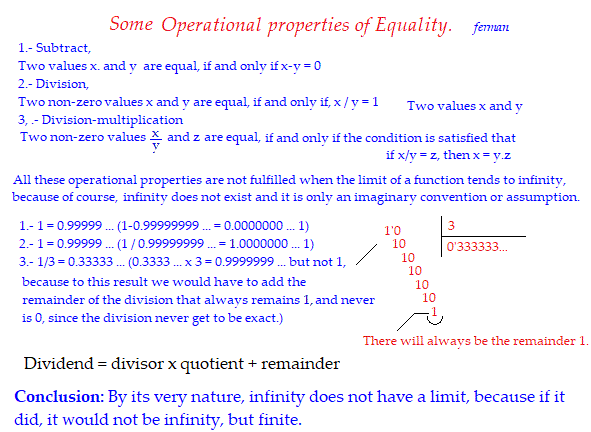
Mastering geometry begins with understanding the essential properties of equalities. Whether you're solving equations, proving theorems, or applying geometric principles, grasping these properties is crucial. In this blog, we’ll explore the fundamental properties of equalities—reflexive, symmetric, transitive, addition, subtraction, multiplication, and division. By the end, you’ll have a clear roadmap to confidently tackle geometry problems. Let’s dive in and unlock the secrets of equalities in geometry,geometry basics,mathematical properties.
What Are the Properties of Equalities in Geometry?

Equalities in geometry are governed by specific properties that ensure consistency and accuracy in mathematical reasoning. These properties are the building blocks for solving equations and proving geometric relationships. Below are the key properties you need to know,geometric principles,equation solving.
1. Reflexive Property
The reflexive property states that any quantity is equal to itself. Mathematically, it’s expressed as:
a = a.
This property is foundational, as it establishes the baseline for all other equality comparisons,basic geometry,mathematical foundations.
2. Symmetric Property
The symmetric property asserts that if one quantity is equal to another, the reverse is also true. It’s written as:
If a = b, then b = a.
This property ensures that equality is a two-way relationship,geometry theorems,equation properties.
3. Transitive Property
The transitive property allows us to chain equalities together. If a = b and b = c, then a = c. This property is essential for multi-step proofs and problem-solving,geometric proofs,mathematical reasoning.
Applying Properties of Equalities in Geometry
Understanding these properties is one thing, but applying them in geometric contexts is where mastery truly shines. Here’s how they’re used in practice,geometry applications,problem-solving strategies.
Addition and Subtraction Properties
The addition property states that if a = b, then a + c = b + c. Similarly, the subtraction property asserts that a - c = b - c. These properties are vital for isolating variables in equations,equation manipulation,geometric calculations.
Multiplication and Division Properties
The multiplication property allows us to multiply both sides of an equation by the same value: If a = b, then a * c = b * c. The division property works similarly: a / c = b / c. These properties are key for scaling and proportion problems,geometric scaling,proportion problems.
📌 Note: Always ensure the value you’re adding, subtracting, multiplying, or dividing by is not zero when applying these properties, especially in division.
Checklist: Mastering Properties of Equalities
- Understand the reflexive, symmetric, and transitive properties.
- Apply addition and subtraction properties to isolate variables.
- Use multiplication and division properties for scaling problems.
- Practice with geometric proofs and equations to reinforce learning.
Mastering the properties of equalities is essential for success in geometry. By understanding and applying the reflexive, symmetric, transitive, addition, subtraction, multiplication, and division properties, you’ll gain the tools needed to solve complex problems and prove geometric theorems. Keep practicing, and soon these properties will become second nature,geometry mastery,mathematical confidence.
What is the reflexive property of equality?
+
The reflexive property states that any quantity is equal to itself, expressed as a = a.
How does the transitive property help in geometry?
+
The transitive property allows chaining equalities, e.g., if a = b and b = c, then a = c, which is crucial for multi-step proofs.
Can you apply the division property if the divisor is zero?
+
No, the division property cannot be applied if the divisor is zero, as division by zero is undefined.


