Understanding Inverse Properties: A Simple Guide

<!DOCTYPE html>
Inverse properties are fundamental concepts in mathematics that help simplify complex equations and expressions. Whether you’re a student, educator, or professional, understanding these properties can significantly enhance your problem-solving skills. This guide breaks down the concept of inverse properties, their applications, and how to use them effectively.
What Are Inverse Properties?

Inverse properties refer to the relationship between two operations that, when applied sequentially, return the original value. The most common inverse properties are found in addition/subtraction and multiplication/division. For example, adding and then subtracting the same number leaves the original number unchanged. Similarly, multiplying and then dividing by the same non-zero number also results in the original value.
📌 Note: Inverse properties are essential in algebra, calculus, and other mathematical disciplines, as they simplify equations and solve for unknowns.
Key Inverse Properties in Mathematics
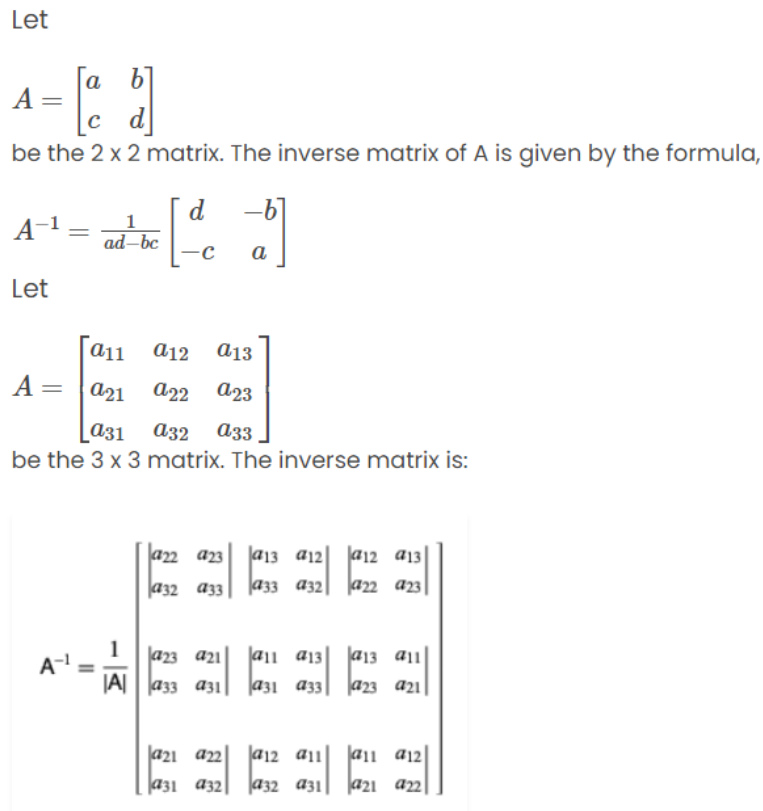
Additive Inverse Property
The additive inverse property states that for any number ( a ), there exists a number ( -a ) such that:
[
a + (-a) = 0
]
This property is useful in solving linear equations and balancing expressions.
Multiplicative Inverse Property
The multiplicative inverse property states that for any non-zero number ( a ), there exists a number ( \frac{1}{a} ) such that:
[
a \times \frac{1}{a} = 1
]
This property is crucial in solving for variables in equations involving multiplication and division.
| Property | Operation | Result |
|---|---|---|
| Additive Inverse | ( a + (-a) ) | 0 |
| Multiplicative Inverse | ( a \times \frac{1}{a} ) | 1 |

Practical Applications of Inverse Properties

Inverse properties are not just theoretical concepts; they have real-world applications in various fields:
- Finance: Calculating interest rates and loan payments.
- Physics: Solving equations related to motion and forces.
- Engineering: Designing structures and systems using mathematical models.
How to Apply Inverse Properties

To apply inverse properties effectively, follow these steps:
- Identify the operation (addition, subtraction, multiplication, or division).
- Determine the inverse operation.
- Apply the inverse operation to simplify the expression or solve the equation.
✨ Note: Always ensure that the number you’re working with is non-zero when applying the multiplicative inverse property.
Checklist for Mastering Inverse Properties

- Understand the definitions of additive and multiplicative inverse properties.
- Practice solving equations using inverse properties.
- Apply inverse properties in real-world scenarios.
- Review and revise regularly to reinforce your understanding.
Mastering inverse properties is a valuable skill that simplifies mathematical problem-solving and enhances your understanding of algebraic concepts. By applying these principles, you can tackle complex equations with confidence and precision. (mathematical properties, algebra basics, equation solving)
What is the additive inverse of a number?
+The additive inverse of a number ( a ) is ( -a ), such that ( a + (-a) = 0 ).
Can the multiplicative inverse property be applied to zero?
+No, the multiplicative inverse property cannot be applied to zero because division by zero is undefined.
How are inverse properties used in real life?
+Inverse properties are used in finance for calculating interest, in physics for solving motion equations, and in engineering for designing systems.



