Understanding Probability Notation: A Quick Guide
Understanding probability notation is essential for anyone working with data, statistics, or predictive modeling. Whether you’re a student, a data scientist, or a business analyst, mastering these symbols and formulas can significantly enhance your ability to interpret and communicate probabilistic concepts. In this guide, we’ll break down the key notations, their meanings, and how to use them effectively.
What is Probability Notation?

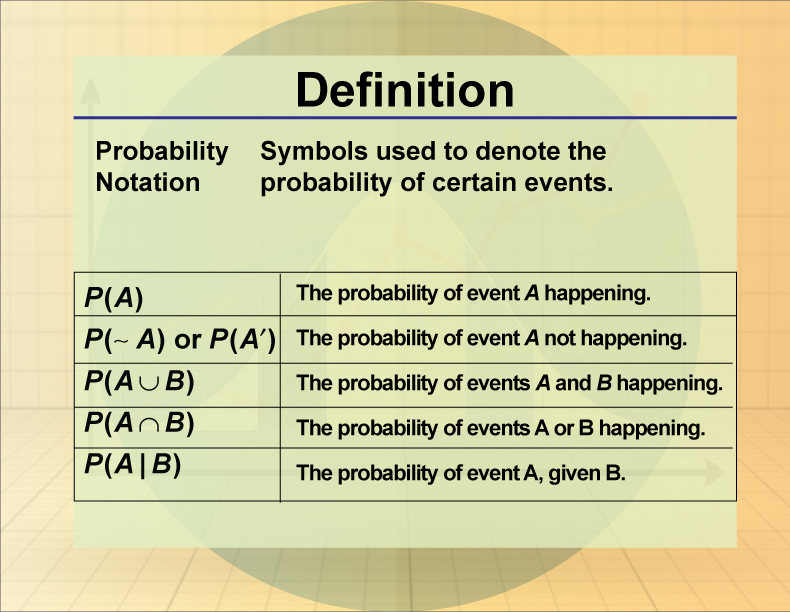
Probability notation is a standardized way of expressing the likelihood of events occurring. It uses mathematical symbols to represent probabilities, making complex ideas easier to understand and work with. Common notations include P(A), P(A | B), and P(A ∩ B), each serving a specific purpose in probability theory.
Key Probability Notations Explained

1. P(A): Probability of Event A
P(A) represents the probability of event A occurring. It is a value between 0 and 1, where 0 indicates impossibility and 1 indicates certainty.
- Example: If A is the event of rolling a 6 on a fair die, P(A) = 1⁄6.
📌 Note: Always ensure the sample space is clearly defined when calculating P(A).
2. P(A | B): Conditional Probability
P(A | B) denotes the probability of event A occurring given that event B has already occurred. It is calculated as P(A ∩ B) / P(B).
- Example: If B is the event of drawing a red card from a deck, and A is drawing a king, P(A | B) = 2⁄26 (since there are 2 red kings out of 26 red cards).
3. P(A ∩ B): Joint Probability
P(A ∩ B) represents the probability of both events A and B occurring simultaneously.
- Example: If A is rolling an even number and B is rolling a number greater than 4, P(A ∩ B) = 1⁄6 (only the number 6 satisfies both conditions).
4. P(A ∪ B): Union Probability
P(A ∪ B) is the probability of either event A or event B (or both) occurring. It is calculated using the formula: P(A ∪ B) = P(A) + P(B) - P(A ∩ B).
- Example: If A is rolling a 1 and B is rolling a 2, P(A ∪ B) = 1⁄6 + 1⁄6 - 0 = 1⁄3.
| Notation | Meaning | Example |
|---|---|---|
| P(A) | Probability of A | P(Rolling a 6) = 1/6 |
| P(A | B) | Conditional Probability | P(King | Red Card) = 2/26 |
| P(A ∩ B) | Joint Probability | P(Even ∩ >4) = 1/6 |
| P(A ∪ B) | Union Probability | P(1 ∪ 2) = 1/3 |

Practical Applications of Probability Notation

Probability notation is widely used in fields like machine learning, finance, and weather forecasting. For instance, conditional probability helps in predicting customer behavior based on past purchases, while joint probability is crucial in risk assessment models.
Checklist: Mastering Probability Notation
- Understand the basics: Familiarize yourself with P(A), P(A | B), P(A ∩ B), and P(A ∪ B).
- Practice with examples: Apply these notations to real-world scenarios.
- Use visual aids: Tables and diagrams can simplify complex calculations.
- Review regularly: Probability notation is a foundation for advanced statistical concepts.
Probability notation, conditional probability, joint probability, union probability, statistical concepts, data analysis, predictive modeling,
What is the difference between P(A) and P(A | B)?
+P(A) is the probability of event A occurring, while P(A | B) is the probability of A occurring given that B has already occurred.
How do I calculate P(A ∪ B)?
+Use the formula P(A ∪ B) = P(A) + P(B) - P(A ∩ B) to find the probability of either A or B occurring.
Why is probability notation important in data science?
+Probability notation provides a standardized way to express and manipulate probabilistic concepts, which are fundamental in data analysis and predictive modeling.
In summary, probability notation is a powerful tool for expressing and understanding the likelihood of events. By mastering P(A), P(A | B), P(A ∩ B), and P(A ∪ B), you’ll be better equipped to tackle complex problems in statistics and data science. Keep practicing, and soon these notations will become second nature!


